TDEs occur when a star, orbiting around a BH, gets disrupted by the BH tides that overwhelm the stellar self-gravity.
Since 1990s, we have been able to see these events via electromagnetic radiation and they have been a powerful tool to unveil the presence of dormant BHs in the cores of galaxies. Yet, we expect them to emit also GWs.
To date, we have not been able to detect their gravitational radiation, since they emit at frequencies lower than the interval where the present interferometers are sensitive. But in the future, when the next-generation interferometers will be operative, we will manage to see these events with GWs. Furthermore, the synergy between new interferometers and telescopes will allow the beginning of the multimessenger era for TDEs.
The scope of this online catalogue is to provide a collection of the gravitational waveforms produced during these events, aiming to be a useful reference for future observations.
If you wish to know more about TDEs, their physical properties, the observations so far and the code used to build this catalogue, please consult the page LINKS.
Basic features of the catalogue
Here we present the catalogue in a schematic way. If you wish to know in more details how we build it, the orbital parameters and the physical parameters considered, please consult Toscani et al. 2021.
The simulations that are part of this catalogue reproduce the first passage of the orbiting star at the pericenter, where the disruption takes place.
The catalogue is now made by 243 simulations. The number of events explored will be updated by the authors during the years, in order to make this catalogue as comprehensive as possible.
These numerical studies are performed with GR PHANTOM, a smoothed particle hydrodynamic code in a general relativistic frame.
The module that we implemted in GR PHANTOM assumes the weak field approximation and the Lorenz Gauge.
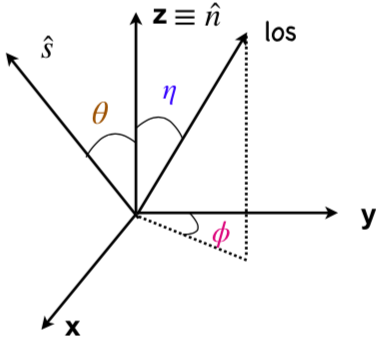
In GR PHANTOM, for spinning BHs, the coordinate choice naturally has the z axis pointing in the BH spin direction. In these cases, we then simulate an encounter with an inclined stellar orbit with respect to the z axis, by an angle θ, and then rotate the results back into the x − y plane before computing the GW emission.
Quadrupole radiation propagating along a generic direction k will depend on two angles. Here, we call these angles η and φ. η is defined as the angle between the line of sight and the z direction, that we will always consider to be perpendicular to the stellar orbit in our TDE calculations. Instead φ is the angle between the projection of the line of sight on the orbital plane and the y axis, along which we will always assume the stellar pericenter to lie in our study. The system of angles is illustrare in the Figure at the bottom of the page.
The waveforms presented here are calculated at 1 Mpc. You can rescale the resuts at the distance you prefer.
We provide the gravitational waveforms at fixed values of (η,φ): (0,0),(30,0),(60,0),(90,0). However, we also offer the components of the second time derivative of the moment of inertia in the x–y plane, so the user can compute easily the GW signal with any geometry.